~~~ La versione in italiano inizia subito dopo la versione in inglese ~~~
ENGLISH
24-11-2024 - Analytic Geometry - Scalar Products [EN]-[IT]
With this post I would like to give a short instruction about the topic mentioned in the subject
(code notes: X_67)
Scalar Products
Scalar products are useful tools to calculate angles and lengths.
Let's consider the standard scalar product on R3.
We will have the following situation

Example
Let's try to calculate the standard scalar product of the following pair of vectors.
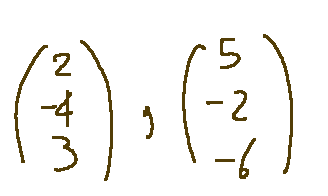
In this case we immediately identify how to calculate the scalar product between these two vectors that we will call V and W.
So the two vectors will be:
v = (v1,v2,v3)
w = (w1,w2,w3)
The scalar product is calculated in the following way:
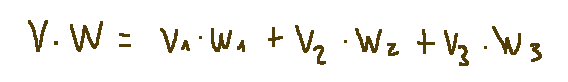
Let's remember what the vectors v and w are like

Let's now proceed with the calculation by substituting the values in the first formula shown in this exercise
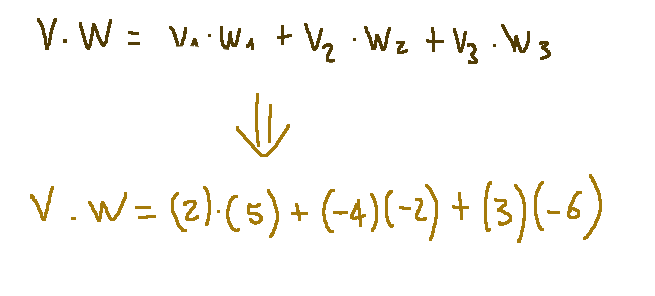
Continuing with the calculations we will arrive at this result
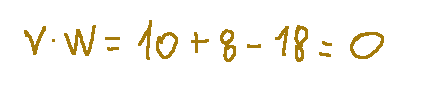
The scalar product will be so
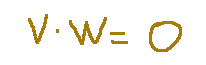
In this case we can say that the vectors are orthogonal
Example 2
Now let's find the standard scalar product of the following pairs of vectors. (1 3 0 -1) and (0 2 -1 4)
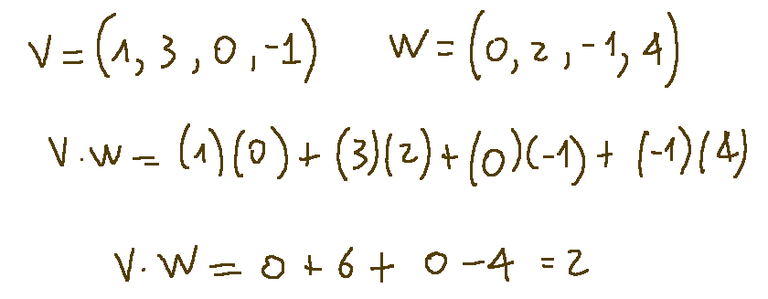
The scalar product is the following
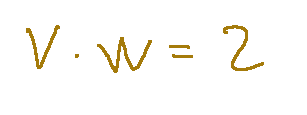
Conclusions
The scalar product between two vectors in a scalar (a number) is the basis of many other mathematical operations.
Question
Have you ever tried to do the scalar product between two vectors?

[ITALIAN]
24-11-2024 - Geometria analitica - Prodotti scalari [EN]-[IT]
Con questo post vorrei dare una breve istruzione a riguardo dell’argomento citato in oggetto
(code notes: X_67)
Prodotti scalari
I prodotti scalari sono strumenti utili per calcolare gli angoli e le lunghezze.
Consideriamo il prodotto scalare standard su R3.
Avremo la seguente situazione

Esempio
Proviamo a calcolare il prodotto scalare standard della seguente coppia di vettori.
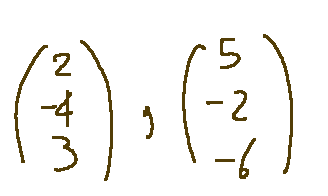
In questo caso identifichiamo subito come calcolare il prodotto scalare tra questi due vettori che chiameremo V e W.
Quindi i due vettori saranno:
v = (v1,v2,v3)
w = (w1,w2,w3)
Il prodotto scalare si calcola nella seguente maniera:
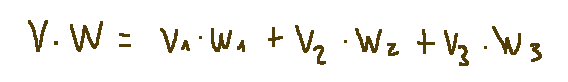
Ricordiamo come sono i vettori v e w

Procediamo ora con il calcolo andando a sostituire i valori nella prima formula mostrata in questo esercizio
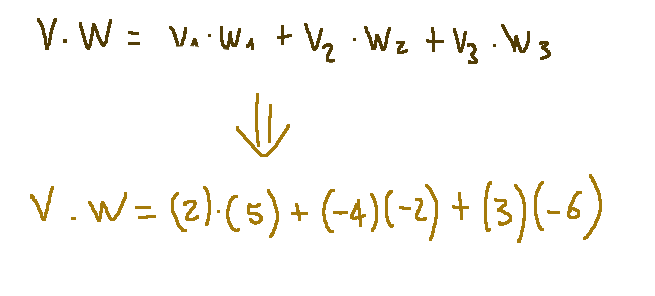
Proseguendo con i calcoli arriveremo a questo risultato
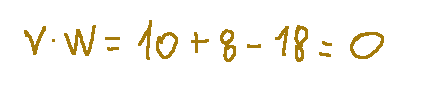
Il prodotto scalare sarà quindi
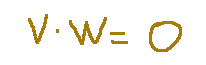
In questo caso possiamo dire che i vettori sono ortogonali
Esempio 2
Troviamo ora il prodotto scalare standard delle seguenti coppie di vettori. (1 3 0 -1) e (0 2 -1 4)
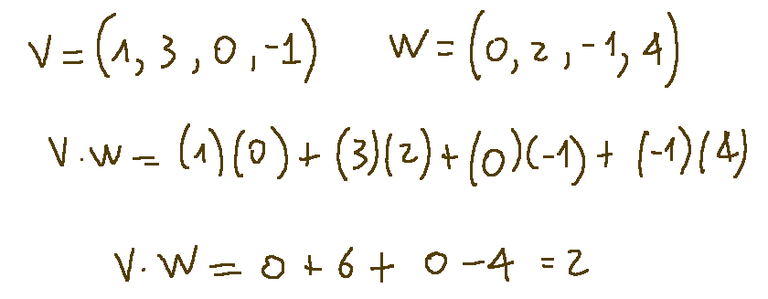
Il prodotto scalare è il seguente
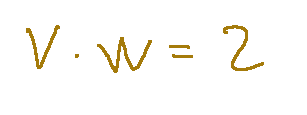
Conclusioni
Il prodotto scalare tra due vettori in uno scalare (un numero) è alla base di molte altre operazioni matematiche.
Domanda
Avete mai provato a fare il prodotto scalare tra due vettori?
THE END




