Cubic Formula Proof Step 3: First Solution of y

mes in #hive-128780 • 5 days ago

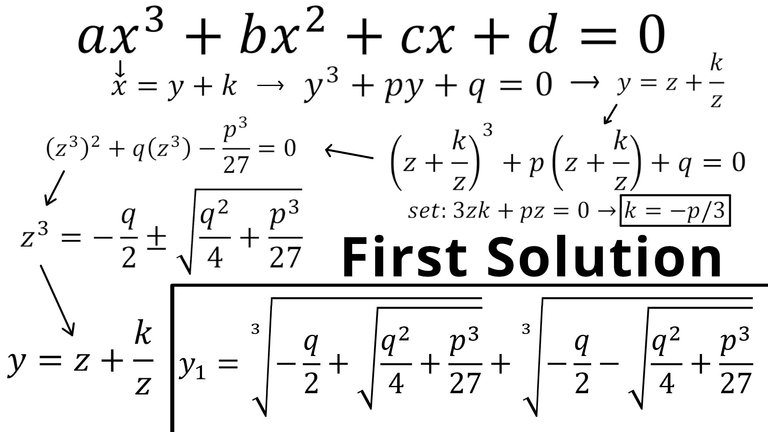
In this video I go over the third step of the Cubic Formula proof which involves plugging our z value back into Vieta's substitution to obtain our first y solution. Recall that in Step 1 we removed the x-squared term from the cubic equation via PQ substitution, and in Step 2 we used Vieta's substitution to obtain a quadratic formula. For Step 3, and since the previous quadratic formula obtained two solutions, we start by plugging in the positive version into Vieta's substitution. After some algebra via conjugate multiplication, we obtain the first solution for y (before we have to plug it into our PQ substitution to obtain x), which has both the positive and negative versions of the quadratic formula solution. This also means that if plugged in the negative version into Vieta's substitution, we would still obtain the positive, thus it doesn't matter which version we plug in!

4 Step 3 First Solution of y.jpeg

Timestamps

Step 3: Find first solution for y using the plus sign for z-cubed and plug into y: 0
Multiply fraction by the conjugate of its denominator to cancel out terms: 3:10
First solution of y: 7:40
The solution obtains both the plus and minus versions of Vieta's quadratic formula: 8:26

Notes and playlists

Playlist: https://www.youtube.com/playlist?list=PLai3U8-WIK0EF05ExjzLbB64NgUjoV1hl
Notes: https://peakd.com/hive-128780/@mes/dzekfnxh .

Become a MES Super Fan! https://www.youtube.com/channel/UCUUBq1GPBvvGNz7dpgO14Ow/join

DONATE! ʕ •ᴥ•ʔ https://mes.fm/donate

SUBSCRIBE via EMAIL: https://mes.fm/subscribe

MES Links: https://mes.fm/links

MES Truth: https://mes.fm/truth
Official Website: https://MES.fm
Hive: https://peakd.com/@mes

Email me: contact@mes.fm

Free Calculators: https://mes.fm/calculators

BMI Calculator: https://bmicalculator.mes.fm
Grade Calculator: https://gradecalculator.mes.fm
Mortgage Calculator: https://mortgagecalculator.mes.fm
Percentage Calculator: https://percentagecalculator.mes.fm

Free Online Tools: https://mes.fm/tools

iPhone and Android Apps: https://mes.fm/mobile-apps

▶️ 3Speak

#math #science #algebra #stemgeeks #chessbrothers #proofofbrain #education #calculus #stem #stemsocial

Sort:

Trending

[-]

mes 5 days ago

!summarize

0.04 VYB

1 vote

[-]

ai-summaries 5 days ago

Part 1/6:

Understanding the Process of Finding Solutions in Algebra

In the discussion surrounding the resolution of a particular mathematical problem, the focus is on identifying the first solution through a structured approach. The process follows a series of well-defined steps, integrating elements of algebraic manipulation and incorporating the principles underlying quadratic equations.

Step-by-Step Approach to Finding the Solution

To find the first solution, the process begins with recognizing the equation in question and identifying the necessary steps. At this stage, we transition into solving for a variable, denoting it as (y), while acknowledging the presence of both a positive and a negative component in our calculations.

Plugging Values into the Equation

0.00 VYB

[-]

ai-summaries 5 days ago

Part 2/6:

Knowing that we need to find (y = \frac{2Z + k}{Z}), the next step involves substituting values into our equation. Here, (k) is defined as ( \frac{p}{3} ), leading us to rewrite (y) as follows:

[ y = \frac{2Z + \frac{p}{3}}{Z} ]

Upon diving deeper into manipulating these equations, we choose to explore the positive side of our calculations first. This decision allows for a more straightforward interpretation of the results, setting the stage for identifying potential conjugate relationships for later use.

Handling the Plus/Minus Factor

0.00 VYB

[-]

ai-summaries 5 days ago

Part 3/6:

One critical aspect of this calculation is the handling of the plus or minus sign present in the radical expressions. Choosing the positive option initially aids in deriving an expression that can later be mirrored through its conjugate. We proceed to find our variable (Z) by taking the cube root, leading to a complex expression which simplifies the calculation process.

Numerous Calculations and Simplifications

0.00 VYB

[-]

ai-summaries 5 days ago

Part 4/6:

As calculations unfold, the systematic plugging back of values into algebraic fractions allows for gradual simplification. Each multiplication and manipulation of the segments leads closer to the first solution denoted as (y_1). By employing algebraic properties, like the ability to multiply by a conjugate, algebraic resources provide the significant reductions necessary to streamline complex expressions.

Finalizing the First Solution

Following comprehensive calculation and reduction, what emerges is an elegant expression for our first solution, (y_1). This expression, thoroughly derived, validates the calculations made through both decomposition and rationalization techniques.

Exploring Alternative Options

0.00 VYB

[-]

ai-summaries 5 days ago

Part 5/6:

Finally, it’s essential to note that if one were to start with the negative option regarding the quadratic formula, the overall results would remain unchanged. This notion emphasizes the robustness of algebraic principles, confirming that regardless of approach—whether selecting the positive or negative side in derivation—the final solution converges to the same end point.

Conclusion

0.00 VYB

[-]

ai-summaries 5 days ago

Part 6/6:

Through this intricate yet systematic process, we have explored the fundamentals of algebraic problem-solving, showcasing the importance of methodical reasoning, simplification through conjugates, and the adaptability of approaches in yielding consistent results. This understanding not only demystifies the mathematical endeavor but also highlights the beauty inherent in algebraic structures and relationships.

0.00 VYB