.jpg)
Position: Position is a measurement that describes an object's location in space at a given time. In physics, a coordinate system is used to describe the position of a particle. The position can be determined by two or three coordinates, depending on the coordinate system used.
Velocity: Velocity is a measure that describes the change in the position of an object in a given time interval. Speed can be expressed in terms of distance traveled and time elapsed. Velocity is a vector, which means it has direction and magnitude.
Acceleration: Acceleration is a measurement that describes the change in velocity of an object in a given time interval. Acceleration can be expressed in terms of initial velocity, final velocity, and elapsed time. Acceleration is also a vector, so it has direction and magnitude.
In physics, the trajectory of a particle can be described using these three aspects: position, velocity, and acceleration. The position of a particle is a function of time, which means that it can be described by a mathematical function. Velocity and acceleration can also be described as functions of time.
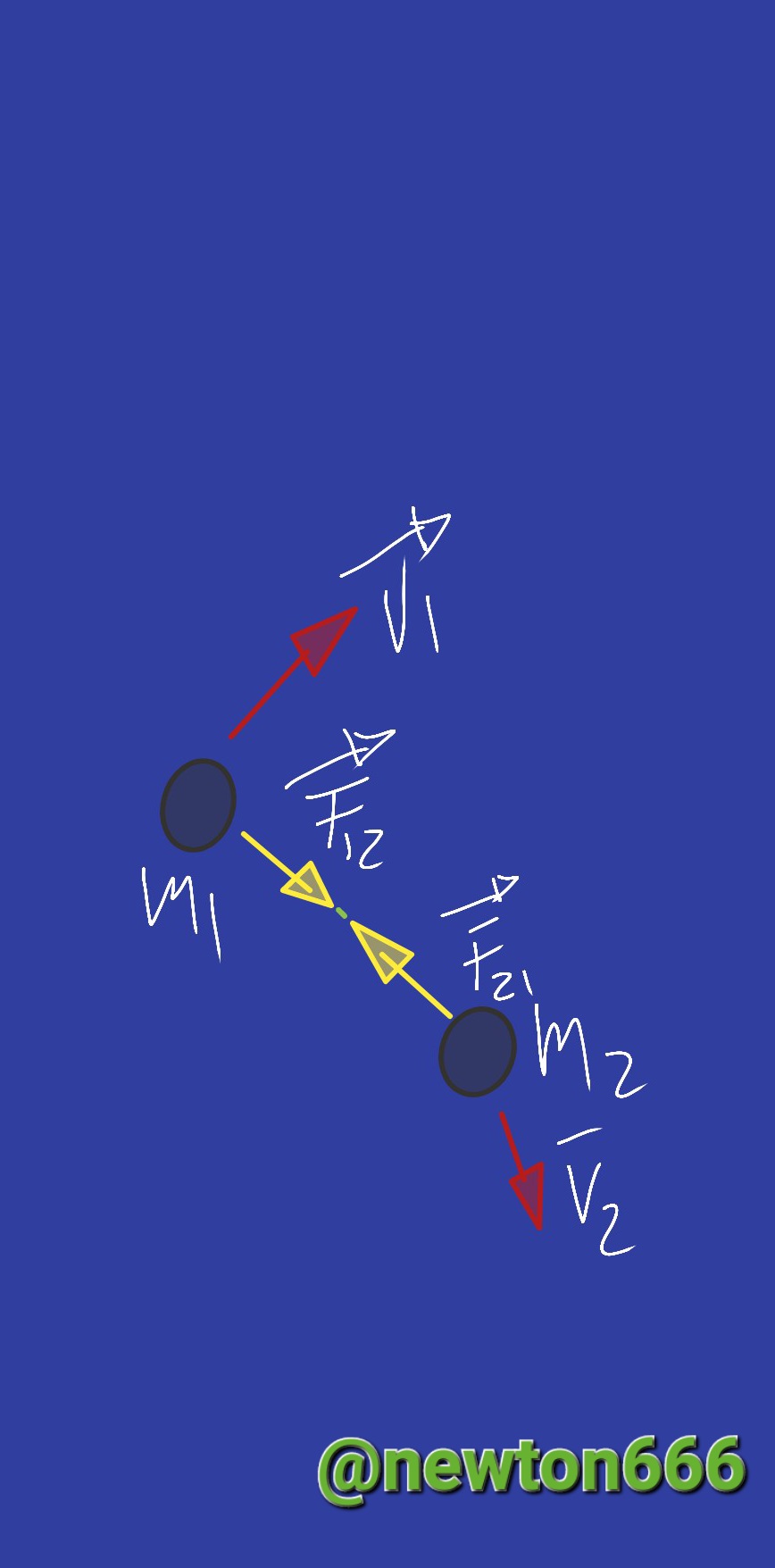
The relationship between position, velocity, and acceleration can be described by Newton's second law, which states that the acceleration of an object is equal to the net force applied to the object divided by its mass. This law allows us to predict the trajectory of a particle by knowing its initial position, velocity and acceleration.
In summary, the trajectory of particles is a fundamental concept in physics, which allows us to understand the movement and behavior of objects in space. The trajectory of a particle can be described using three aspects: position, velocity, and acceleration. These aspects can be described as functions of time and are related.
Exercise 1:
Suppose that a particle moves in a curved path and that the position of the particle at any moment can be described by the function:
x = 2t^3 - 6t^2 + 4t
y = 3t^2 - 4t + 2
Where x and y are the coordinates of the particle and t is the time.
a) Find the velocity of the particle at t = 2 seconds.
b) Find the acceleration of the particle at t = 2 seconds.
Solution:
a) The velocity can be found by differentiating the position function with respect to time:
vx = 6t^2 - 12t + 4
vy = 6t - 4
Evaluating these functions at t = 2, we have:
vx = 6(2)^2 - 12(2) + 4 = 12 - 24 + 4 = -8
vy = 6(2) - 4 = 8 - 4 = 4
The speed of the particle at t = 2 seconds is (-8, 4) m/s.
b) Acceleration can be found by differentiating the velocity function with respect to time:
ax = 12t - 12
ouch = 6
Evaluating these functions at t = 2, we have:
ax = 12(2) - 12 = 0
ouch = 6
The acceleration of the particle at t = 2 seconds is (0, 6) m/s^2.
Exercise 2:
A particle moves in a curved path and the position of the particle at any time can be described by the function:
x = 2cos(t)
y = 3sin(t)
Where x and y are the coordinates of the particle and t is the time in radians.
a) Find the velocity of the particle at t = π/4 radians.
b) Find the acceleration of the particle at t = π/4 radians.
Solution:
a) The velocity can be found by differentiating the position function with respect to time:
vx = -2sin(t)
vy = 3cos(t)
Evaluating these functions at t = π/4, we have:
vx = -2sin(π/4) = -√2
vy = 3cos(π/4) = 3√2
The velocity of the particle at t = π/4 radians is (-√2, 3√2) m/s.
b) Acceleration is a vector quantity that measures the rate of change of the velocity of an object. The unit of measurement is the meter per second squared (m/s^2). The direction of acceleration is the same as the direction of change of velocity.
Exercise 1:
An object moves with a constant speed of 10 m/s for 2 seconds. What is its acceleration?
Solution:
Since the velocity is constant, the acceleration is equal to zero.
Exercise 2:
An object moves with an initial velocity of 10 m/s and accelerates at a rate of 2 m/s^2 for 3 seconds. What is its final velocity?
Solution:
We can use the formula v = v0 + at to calculate the final velocity, where v is the final velocity, v0 is the initial velocity, a is the acceleration, and t is time.
v = 10 m/s + (2 m/s^2) * 3 s = 10 m/s + 6 m/s = 16 m/s
Therefore, the final velocity is 16 m/s.
Solution:
We can use the formula v = v0 + at to calculate the final velocity, where v is the final velocity, v0 is the initial velocity, a is the acceleration, and t is time.
v = 10 m/s + (2 m/s^2) * 3 s = 10 m/s + 6 m/s = 16 m/s
Therefore, the final velocity is 16 m/s.
Thanks to these two examples, two examples show how position, velocity and acceleration are interrelated and how they can be calculated using basic physics formulas. Understanding these concepts is fundamental to the study of dynamics and mechanics.Thanks to these two examples, two examples show how position, velocity and acceleration are interrelated and how they can be calculated using basic physics formulas. Understanding these concepts is fundamental to the study of dynamics and mechanics.
Photo edited by my Samsung A23 phone
Bibliography Reference
University Physics by Francis Sears and Mark Zemansky, 2002.
Classical Mechanics by Herbert Goldstein, 1980.
An Introduction to Mechanics by Kleppner and Kolenkow, 2002.


