Newtons 3rd law of motion and conservation of momentum

Introduction

Yesterday, I looked at Newtons 2nd law of motion which tries to explain what happens to a body when an external force acts on it. The three quantities evaluated are force, mass and acceleration. If you wish to learn more about that law, here is the post. Today, I wish to look at the 3rd law and conservation of Linear momentum.

So lets start by stating Newton's 3rd law of motion. Here is it:

For every action, there is an equal and opposite reaction.source

Understanding Newton's 3rd law of motion

With this 3rd law of motion, Newton tries to explain the nature of forces between two interacting bodies. When a body exerts a force on another object, the object also returns some force against the first body. Forces always operate in pairs. When a force is exerted on a body which could be considered an "action", the body also produces its own force which is considered a "reaction". These two forces always exist and acts in pairs on colliding or interacting bodies.

The third law goes on to explain the magnitude of the action and reaction forces. The acting force and the reacting force are said to be of the size or magnitude. And when it comes to the direction, they operate in opposite directions. If the acting force moves along south, the reacting force should operate in the direction of the north - and vice versa.

Newtons 3rd law of motion is fundamental to understanding how objects move and other kinds of motions on the earths surface. Lets see so real examples of the action-reaction forces as explained by the third law.

3rd law example

We can see the 3rd law in action in various motion activities around us. Consider the following 4 examples:

• Recoil of a gone: When a bullet is fired from a gone, the bullet moves forward. But while it is leaving the gun, it exerts its own force on the gun by suddenly pushing it backwards too. The bullet fired is the action force, the bullet pushing the gun barrel backwards is the reaction force.

• Vehicle Collision: When two vehicles collide, both gets damaged. Whether a moving vehicle collides with a stationary vehicle, or two moving vehicles collide with each other. Both vehicles get damaged because the exact an equal and opposite force on each other.

• How a fish swims: A fish swims by pushing the water backwards with its fins. That is the action force. The water reacts by push the fin and fish forward. Through this action and reaction forces, fish can move through water easily.

• How we walk: When a person walks, they use their foot to push the ground backward. The ground also produces its reaction force by pushing the person forward. In this way, a person can walk on the ground and move forward.

These are just a few of the many examples of action-reaction forces that we observe around us. We can use these to understand that Newtons third law has a fundamental application everyday.

Principle of Conservation of Linear momentum

From Newton's second and thirds laws of motion, we draw the principle of conservation of Linear momentum. This principle helps us understand the nature of forces in two bodies before and after collision.

Below is how the principle is stated:

the principle of conservation of momentum states that when you have an isolated system, the initial total momentum of objects before a collision equals the final total momentum of the objects after the collisionsource

This principle only applies to an isolated system. That means there are no other external forces acting on the colliding bodies. If that is the case, then the momentum in such a system is conserved. Simply put, when two bodies collide, their moment after collision is the sum total of each of their momentums before collision, provided there are no external influences like a push or vibration on the system.

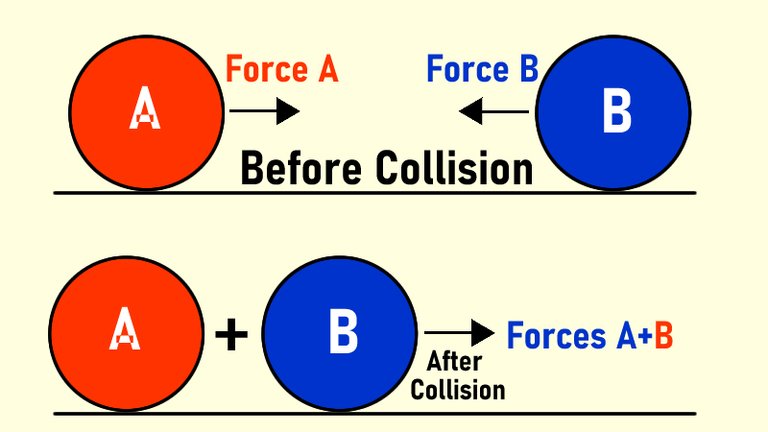
Considering the two objects above A and B. Each has its momentum before collision. After collision the two forces join to become one. The size of the momentum after collision is AB, which the sum of each objects momentum A and B before collision. Lets see an example.

Worked example

A bullet is fired into a block of wood and both move with a velocity of 0.7ms^-1 after collision. if the bullet has a mass of 0.0
7g and the wood has weight of 13kg, find the velocity of the bullet before it hit the wood.

Solution

Let the initial velocity be V ms^-1
Momentum = initial velocity X mass = 0.07 x V
Momentum after collision = 13x0.7

Principle of conservation of momentum show that before collision and after collision, momentum is conserved. So

13 x 0.7 = 0.07 x V
V = 13 x 0.7/0.07
V = 130 ms^-1

Conclusion

Newton's 3rd law of motion helps us understand how bodies react during collision. It also shows that forces work in pairs. The principle of conservation of momentum also makes it clearer to understand the state of forces within a closed system of colliding bodies.

Reference materials

_{Newton’s laws of motion}

_{Principle of Conservation of Momentum}

_{Newton's Third Law}

All Images are mine.