Dark matter signals at CERN’s Large Hadron Collider

In the blog of this week, I decided to change a little bit the topic, and to focus on one of the darkest and more secret substances in our universe. Obviously, this post is not about the darkest secrets of Dumbledore or Grindelwald, or anything related to them (apologies… but I had to make that joke; I saw the movie a few weeks ago). It is all about physics and … dark matter (and thus not on wizardry).

Dark matter is one of the greatest motivation for the existence of a theory beyond the Standard Model of particle physics, and it is therefore searched for… well… mostly everywhere. To mention some examples (for crispy details, please see here), cosmic rays are deeply probed with satellites; direct detection experiments patiently wait to monitor some dark matter particle playing some snooker with an atomic nuclei; and particle colliders are trying to directly produce dark matter particles in high-energy collisions.

In the present post, I focus on the latter option, and in particular on the best ways to model dark matter production at CERN’s Large Hadron Collider (the LHC). As usual, those pushed for time or not ready to get a full shot of physics can grab the essence of this blog by finishing to read the present section, before jumping directly to the post’s summary. For the others, please grab a pizza (it was Bitcoin Pizza Day recently) and let’s move on with (hopefully) exciting dark news!

So, what does ‘modelling dark matter production at the LHC’ mean? This is not about the ways dark matter is searched for at the LHC, but more about how dark matter signals should be modelled. This is important to make sure we come up with the best possible strategy to look for dark matter in LHC data, and to compare data with theory for conclusive statements.

In practice, we don’t know what dark matter is. Particle physicists therefore came up with hundreds of theories, models and interesting setups. However, in terms of LHC signals, it is better to run away (hundreds of models to consider is just too scary), and consider minimal models instead. In a minimal model, we minimally modify the Standard Model to get a theoretical framework that could accommodate a dark matter particle which can be produced at the LHC (and nothing else).

Minimality has a huge advantage over anything else: it is minimal (I am sure you didn’t guess that)! This means that with a very limited number of free parameters, we can describe LHC dark matter signals and design very good strategies to find them. In a second step, we can relate minimal models to full theories. The interesting point is that one single minimal model has a connection with many complete models (one stone, many birds).

^{[Credits: Original image by ESA/NASA (CC BY 4.0)]}

However, it has been recently pointed out by several groups of researchers that those models may be in fact over-simplified… A big disadvantage of minimal models is therefore… that they are minimal! They are too far from real models of dark matter, and some useful handles on signals could be missed because of that. Minimality is thus both good and bad at the same time…

This blog discusses one of my recent research works in which collaborators and I focused on this minimal/non-minimality issue. In our scientific article, we have shown that in spite of all the complications arising from following a non-minimal path, there is a systematic strategy that could be considered. The key point is to pick a modelling lying not too far from minimality, but exhibiting next-to-minimal features. This strategy then leads to search options non present in minimal models, and that can be exploited in dark matter searches.

In the rest of this blog, I first summarise in a few lines what dark matter is, why physicists believe it is interesting and how we search for it at particle colliders. Next I move on with the modelling of dark matter signal, minimally and non-minimally, and I finally discuss some of the results that I found together with my collaborators.

---

Why dark matter at all?

---

As said above, dark matter is everywhere. But as a matter of fact this is not a fresh news from yesterday. The core idea is actually almost 100 years old. In the 1930s, a Swiss physicist named Zwicky studied how stars rotated inside galaxies. He verified whether this was in agreement with Newton’s gravity and everything that was visible in the sky.

The answer was quite surprising, and the so-called galaxy rotation curves demonstrated that stars were rotating too fast. Their motion could however be explained by theory after assuming the presence of some invisible matter. This invisible matter was thus only noticeable through its gravitational effects, and allowed to restore agreement between theory and data for the motion of stars in galaxies. Dark matter was born. A couple of decades later, this possibility was more deeply confirmed by Rubin.

The universe has thus dark secrets! And these secrets are gravitationally interacting! This consisted only of the first of many (indirect) signs pointing to the possible existence of dark matter.

^{[Credits: Pablo Carlos Budassi (CC BY-SA 3.0)]}

Let’s now move on with a second convincing sign that dark matter could be there.

From the 1960s to today, we got a better and better understanding of something called the cosmic microwave background. In standard cosmology, atoms formed 380,000 after the Big Bang. This means that at this exact moment, the universe went from a soup of electrically-charged particles to a soup of neutral atoms.

This has a very important consequence for electromagnetic radiation. As the latter interacts with anything electrically charged, it could suddenly travel the universe across long distances (no more charged beasts to trip it up). This radiation (called the cosmic microwave background) is thus mostly still there today.

The important point is that it carries an imprint of how the universe was in 380,000 A.B. (after Big Bang). Precision cosmology tells us that this imprint is crystal dark: we need dark matter.

Te story is however not finished. There are additional signs that dark matter exists (although indirect, they are quite convincing). For instance (I quote a last one and then I move on), we can try to explain the formation of the large structures in the universe (galaxies, clusters and super-clusters of galaxies). The conclusions of any simulation that we may want to run are very dark, once again. Without dark matter, gravity is not powerful enough to get where we are today.

Therefore, the universe as it is today would not result from the big bang without dark matter. Crazy, isn’t it? We thus know that dark matter could exist through numerous indirect evidence. However, we have no idea about what dark matter is (for that reason, no-dark-matter alternatives are still considered). Whereas I like pink unicorns, hundreds of other ideas are possible. And this is where it becomes tricky. How to account for all those possibilities in searches for dark matter?

Whereas there are several ways to look for dark matter, I focus in the following on dark matter at the LHC.

^{[Credits: CERN]}

---

Dark matter at particle colliders

---

In the following, we assume that there exists a dark matter particle, and that this particle interacts with the Standard Model of particle physics. In this case, dark matter can be produced in high-energy collisions. Otherwise, life at particle colliders won’t be very dark, and will thus be less interesting.

We know since the time of Einstein that mass and energy are two incarnations of the same thing (more precisely, mass is just one form of energy among others). Therefore, we can produce massive particles (like dark matter particles) in a highly energetic collision.

In such a collision, we accelerate particles to incredibly high speeds, so that their resulting kinetic energy can be converted into mass energy. This simple mechanism opens the door to producing new particles never observed so far. Whereas energy is always conserved in any physical process (that’s an incontrovertible golden rule), energy can always be converted from one form to another. In other words, we can produce massive dark matter particles at colliders through the conversion of kinetic energy into mass.

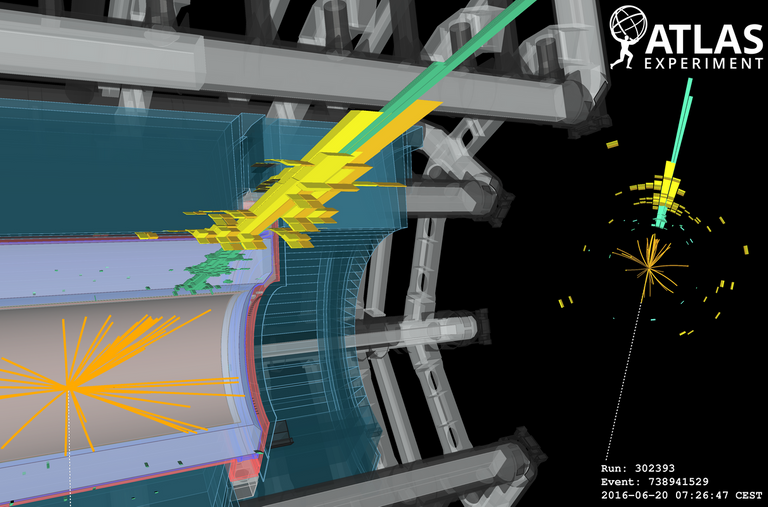
But what is the signature of a dark matter signal? This is illustrated both in the figure below and in the figure above.

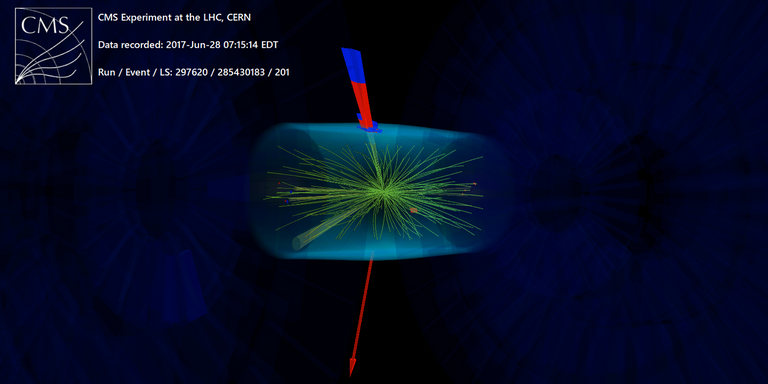
^{[Credits: CERN]}

The detection of dark matter relies on energy and momentum conservation. The same golden rule as that mentioned above pleads again guilty. Additionally, we need to account for the fact that dark matter is super-weakly interacting. This means that once produced, dark matter stealthily escapes any particle detector without leaving any track in it. It is thus not trivial to ’see it’. In other words, the detection of dark matter is equivalent to seeing something invisible.

Whereas this does not sound easy, energy conservation saves us. Let’s explain this on the example of the above figure.

Before the collision, the sum of all energies and momenta are all located along the collision axis (i.e. along the beams). In the initial state of the collision, there is nothing except for the beams that are aiming at each other. In particular, there is nothing in the transverse plane (i.e. there is no particle moving transversely to the beams).

We however observe phenomena in the plane transverse to the beams after the collision. The main reason is that we don’t want to have the colliding beams in the way. Therefore, the sum of the energies and of the momenta in the transverse plane must be zero after the collision, exactly like before the collision. Energy and momentum are conserved, after all!

In the above figure, the transverse energy deposits are shown in red and blue, and we see particle tracks as green lines. Doing the calculation, we notice that something is missing for energy and momentum conservation to work. It is represented by the red arrow in the lower part of the figure. This arrow is hence associated with some particles leaving the detector invisibly, and thus possibly dark matter (or some neutrinos of the Standard Model).

This brings us to the definition of a dark matter signal at particle colliders: we need some large amount of missing transverse energy and a bunch of visible particles. The visible stuff is needed for the detector to trigger and record the picture of the collision. I now move on with the next question: how to model those signals?

^{[Credits: CERN]}

---

Minimal and next-to-minimal-minimal dark matter

---

As we don’t know what dark matter is, there are gazillions of models to consider. Moreover, each model comes with many free parameters that can vary freely. This leads us to a real problem: resources. We don’t have enough resources to take care of simulating all those models and test them against data individually. For that reason, physicists designed simplified (or minimal) models that should reproduce the collider phenomena associated with many dark matter models at once.

Simplified models are built in a very simple way.

• We start from the Standard Model and keep it as such.
• We include a dark matter particle and make it stable thanks to some symmetry (otherwise, if dark matter is unstable then there won’t be dark matter anymore in our universe).
• We connect the dark matter particle to the Standard Model via a mediator relating them.

That’s all. This minimal construction leads to a theoretical framework containing two new particles and their limited set of interactions (i.e. the connection between dark matter, the mediator and the Standard Model).

The most considered specific case is when the Standard Model particle to which dark matter is connected is a quark. In this case, the model predicts a mono-jet signal of dark matter. This means that dark matter is mostly produced in association with a jet of strongly-interacting particles (see here for more information on what those jets are).

The next step is then to reinterpret the experimental results in the framework of any of the numerous complete models of dark matter.

However, simplified models suffer from one big problem: they may not be sensitive to all signals of dark matter that could be encompassed in a complete model. In addition, they also feature a bunch of theoretical inconsistencies due to the fact that they are incomplete by definition.

^{[Credits: NASA/ESA (CC BY 2.0)]}

With my collaborators, we have tried to see what could be done to solve that problem. We investigated an example. We choose a model in which dark matter connects to the Standard Model via a scalar particle, i.e. a particle without spin like the Higgs boson. In a second step, we tried to clear a bit of the inconsistencies of the chosen simplified model. I won’t enter into details, but feel free to ask in the comment section of this blog.

The conclusion is that this led us to a next-to-minimal model in which several mediators were present, with a small set of interactions with the Standard Model. Such a modification has been found to be sufficient to better mimic the entire dark side of complete models. For what concerns the LHC, it turned out that on top of the mono-jet signal that was already predicted in the minimal case, two extra collider signals arose, like in complete models in which it is very rare that there is only a single dark matter signal.

In the figure below, I show an example in which all these new handles on dark matter speak with each other.

^{[Credits: arXiv:2110.15391]}

In this illustration of the phenomenology relevant for next-to-minimal models, we vary the mass of the lightest of the mediators (that’s the X-axis) and fix the mass of all other particles (for the sake of the example). The coupling of this lightest mediator to the Standard Model is shown on the Y-axis.

First of all, we can find below the flashy green curve all scenarios in which we don’t have too much dark matter in the universe (so that they are allowed). In addition, the dark green region is that in which we don’t have too much dark matter contributions to cosmic rays, relative to observations. Those dark green points are thus those we should test as much as possible as very motivated by cosmology.

Second, the red, blue and dark contours are the points excluded by the LHC. The grey region is excluded by standard mono-jet searches (like in the minimal model). In red, we have constraints coming from the production of dark matter with a Higgs boson (i.e. a mono-Higgs probe). Finally the blue region is excluded by constraints coming from the production of dark matter with a pair of top quarks.

We can observe a complementarity between the various sets of constraints: they target different parts of the figure. Moreover, some of the interesting scenarios (the dark green points) are already reachable at the LHC with current data (using mono-Higgs probes). This means that with future data, those next-to-minimal models will become more and more covered, which will yield more hints on what dark matter could be and could not be.

---

Summary: complementary non-minimal dark matter signals at the LHC

---

This new blog is about dark matter and how to model associated signals at particle colliders like the LHC at CERN.

Dark matter is very motivated by various cosmological observations, and it is thus actively searched for. One exemple concerns dark matter searches at the LHC. Usually, the latter are interpreted in the framework of minimal simplified models that are constructed to represent many full models of dark matter at once. This follows the ‘one stone many birds’ picture.

However, while very powerful, minimal models have been found to be problematic in the sense that they do not capture all possible handles on dark matter (from the perspective of the variety of handles that arise from a complete model). In addition, simplified models are often inconsistent theoretically.

This is the problem which I investigated recently with a few collaborators. We demonstrated that curing the theoretical issues inherent to a given set of simplified models for dark matter naturally leads to a next-to-minimal model that offers a better framework to connect with many complete models for dark matter.

We have shown that in such a next-minimal framework, several LHC signals collaborate with each other and help us to probe dark matter better, which provide thus better handles in the light of a future detection. This provides hence a new pathway for studying dark matter at colliders.

I believe it is time to stop writing for today. I am available for comments, questions and remarks, as usual. Please do not hesitate to ask anything about this blog, or about particle physics and cosmology in general.

If you are in addition interested in a citizen science project on Hive, feel free to check out this blog and the #citizenscience tag. I will release the answer to the last assignments by the end of the week.