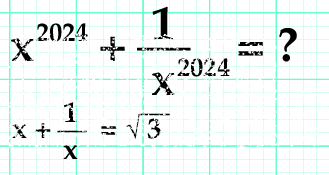| Español | English |
Enunciado
x + 1/x = √ 3 , |
|---|
Este tipo de problemas pertenecen al ámbito de las funciones simétricas y aunque es posible solucionarlos de una forma algebraica , vamos a seguir la senda del análisis combinatorio .
Preliminares
x = α , 1/x = β
-a1 = (1) = ∑ α = α + β = √ 3
a2 = (12 ) = ∑ α β = α ∙ β = 1
Nos facilitan los valores de las funciones simétricas elementales (1), (12).
Nos piden determinar el valor de la suma de potencias de índice 2024.
s2024 = (2024) = ∑α 2024 = α 2024 + β 2024
Desarrollo
La relación entre las funciones simétricas elementales y la suma de potencias es la razón de ser de las identidades de Newton ,
sn − a1sn-1 + a2sn-2 − ... + (−1)nnan = 0
Esta ecuación nos permite establecer una relación de recurrencia en sn
s1 = a1
...
sn = a1sn-1 − a2sn-2 + ... + (−1)n + 1nan
s1 = − √ 3
s2 = (− √ 3 ∙ − √ 3 ) − (2 ∙ 1) = 1
an = 0 , n > 2
sn+2 = − √ 3 ∙ sn+1 − sn
Hemos obtenido una recurrencia de orden dos, su ecuación característica determina el término general de la sucesión,
p2 + √ 3 p + 1 = 0
Con soluciones,
p = e ± i ∙5/6∙π
De la naturaleza de las soluciones de la ecuación característica inferimos que la sucesión es periódica,
5/6 ∙ n = 2k
5n = 12k
n = 12
∴ sn+12 = sn
sn+6 = -sn
Resolución
Solución
2024 ≡ 8 mod 12
s2024 = s8 = - s2
s2024 = - 1
∎
| English | Español |
Statement
x + 1/x = √ 3 , |
|---|
This is a problem on symmetric functions , solvable by pure algebraic methods, but here we are taking the combinatorial route and seeing...
Prelude
x = α , 1/x = β
-a1 = (1) = ∑ α = α + β = √ 3
a2 = (12 ) = ∑ α β = α ∙ β = 1
We know value of the elementary symmetric functions (1), (12).
We are asked for the value of the powers sum with 2024 index .
s2024 = (2024) = ∑α 2024 = α 2024 + β 2024
Development
Symmetric elementary functions related to powers sum ones by means of the Newtonian identities ,
sn − a1sn-1 + a2sn-2 − ... + (−1)nnan = 0
That is a recurrence relation on sn ,
s1 = a1
...
sn = a1sn-1 − a2sn-2 + ... + (−1)n + 1nan
s1 = − √ 3
s2 = (− √ 3 ∙ − √ 3 ) − (2 ∙ 1) = 1
an = 0 , n > 2
sn+2 = − √ 3 ∙ sn+1 − sn
We have a recurrence of second order, the general term is formed with solutions of its characteristic equation ,
p2 + √ 3 p + 1 = 0
p = e ± i ∙5/6∙π
We can infer that the sequence is periodic as the solutions of its charaterisitic equation .
5/6 ∙ n = 2k
5n = 12k
n = 12
∴ sn+12 = sn
sn+6 = -sn
Solution
Answer
2024 ≡ 8 mod 12
s2024 = s8 = - s2
s2024 = - 1
∎
Media